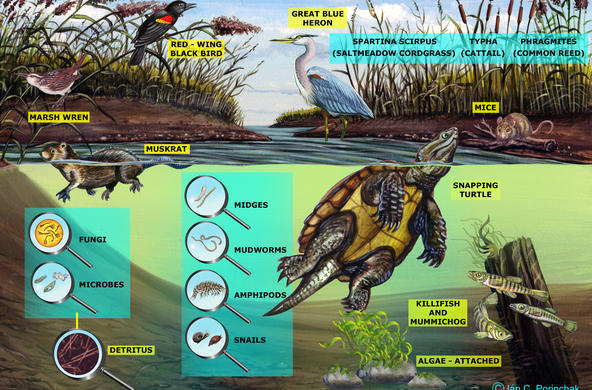
Objectives
Models can be created to represent complex aspects of the real world. Scientists use models to study complex real world situations.
Overview
Rating:
Students will make a three-dimensional candy model of their school using their sketches as a guide.
Materials
(per pair of students)
- 8.5 X 11 sheet of paper glued to a piece of cardboard
- Glue
- Student sketches of the school
- Colored candies (6 colors, 18 oz candy/student pair)
- Pencils
Procedure
- Explain to the class that the they will be using their sketches to make a model of their school grounds using different colored candies. The candy is for the model, NOT for eating! Have the students try to imagine what a miniature version of their school would look like, and some of the key features that must be included, such as trees, buildings, etc.
- Hand out the cardboard with grid sheets, and pencils. The 8.5 X 11 paper represents the school property. Have the students mark with pencil where on the paper they believe key features should go. An example on the chalkboard will aid with this. Did they remember play fields and lawns? Once they have a plan they can proceed to the next step.
- Pass out the candies and glue, and have the students choose what type of feature each color should represent. Examples are: Lawn, cement, road, buildings, trees, etc. Be aware that there are only six colors. Each pair of students should design a color key before they start creating the model. (See student handout.)
- Students use the candies and glue to create a 3-dimensional model of their schoolyard.
- Ask the students how accurate they believe their models are, and what someone new to their school might learn from looking at such a model.
Resources
Lesson Files
pdf
Candyland Model Handout